Mastering Quadratic Equations with Ease: A Comprehensive Guide
페이지 정보

본문
Factoring Quadratic Equations
Factoring quadratic equations involves expressing the equation as the effect of two binomials. This method is helpful when the quadratic equation can be without difficulty factored, but it can be challenging when the equation does not piece easily. To factor a quadratic equation, we necessity to on two numbers whose product is ac and whose sum is b. If you beloved this write-up and you would like to acquire a lot more details pertaining to Quadraticequationcalculator.Com kindly stop by our own web-site. These numbers are the coefficients of the two binomials. After criterion, the quadratic equation x^2 + 5x + 6 can be factored as (x + 3)(x + 2), where the coefficients of the two binomials are 3 and
Algebra: Math calculators can be in use accustomed to to elucidate linear equations, quadratic equations, and other types of algebraic equations.
Calculus: Math calculators can be used to figure out calculus problems, such as derivatives and integrals.
Geometry: Math calculators can be habituated to to reveal geometry problems, such as crafty the area and verge of shapes.
Trigonometry: Math calculators can be employed to untangle trigonometry problems, such as calculating the sine, cosine, and tangent of angles.
Applications of Quadratic Equations in Real-World Scenarios
Quadratic equations get numerous applications in real-world scenarios. In physics, they are acclimated to to describe the motion of objects at the beck constant acceleration, such as the track of a ball thrown upwards or the signal of a buggy accelerating down a level road. In engineering, quadratic equations are used in the intention of electronic circuits, bridges, and buildings. In economics, they are adapted to to model the behavior of markets and the effect of behaviour changes on remunerative variables. The power to clear up quadratic equations efficiently is, the case, essential in the interest professionals and students in these fields, and a Quadratic Equation Adding machine can be a valuable resourc
Understanding the Significance of Quadratic Equations
The import of quadratic equations lies in their capacity to model real-world situations. Seeing that case in point, the trajectory of a missile subservient to the pull of severity can be described through a quadratic equation. Similarly, quadratic equations are reach-me-down in electrical engineering to analyze circuits, in computer science as a service to algorithm conception, and in economics to model deliver and request curves. Donn‚e the intricacy of solving these equations manually, particularly when dealing with adipose numbers or when the equation does not consideration readily, a Quadratic Equation Calculator can be an urgent tool. It not only simplifies the development of pronouncement solutions but also reduces the chance of altruist foul-up, providing error-free results instantl
Benefits of Using a Quadratic Equation Calculator
Using a Quadratic Equation Adding machine has a sprinkling benefits, including precipitousness, exactness, and convenience. These calculators can explain quadratic equations post-haste, eliminating the necessity in place of manual calculations and reducing the jeopardy of errors. Additionally, they can traffic in complex equations with ease, making them an chief implement in requital for students and professionals. Furthermore, online resources and software provide interactive lessons, examples, and exercises to expropriate users understand the underlying concepts and realize the potential of problem-solving skill
Mastering Quadratic Equations: A Wide Guide
Introduction to Quadratic Equations
Quadratic equations are a constitutional concept in mathematics, and they have numerous applications in various fields such as physics, engineering, and economics. A quadratic equation is a polynomial equation of extent two, which means the highest power of the fickle is two. It has the general body of ax^2 + bx + c = 0, where a, b, and c are constants, and x is the variable. Solving quadratic equations can be challenging, but with the facilitate of tools like the Quadratic Equation Calculator, it can ripen into much easier. In this article, we will delve into the sphere of quadratic equations, analyse their properties, and review various methods on solving the
The Quadratic Formula
The quadratic formula is a powerful machine suitable solving quadratic equations, and it's extensively second-hand in mathematics and science. The quadratic modus operandi is conceded past x = (-b ± v(b^2 - 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic equation. This method provides two solutions to the equation, and it's key to appreciate the concept of discriminant (b^2 - 4ac) to arbitrate the nature of the solutions. If the discriminant is positive, the equation has two patent real solutions. If the discriminant is zero, the equation has one repeated real solution. If the discriminant is adversative, the equation has two complex solution
Arithmetic calculations: These suggest the use of basic arithmetical operations, such as joining, subtraction, multiplication, and division.
Algebraic calculations: These involve the avail oneself of of variables and mathematical operations to solve equations and inequalities.
Geometric calculations: These incorporate the use of exact operations to interpret problems mutual to shapes and spaces.
Trigonometric calculations: These mean the press into service of arithmetical operations to solve problems coordinated to triangles and wave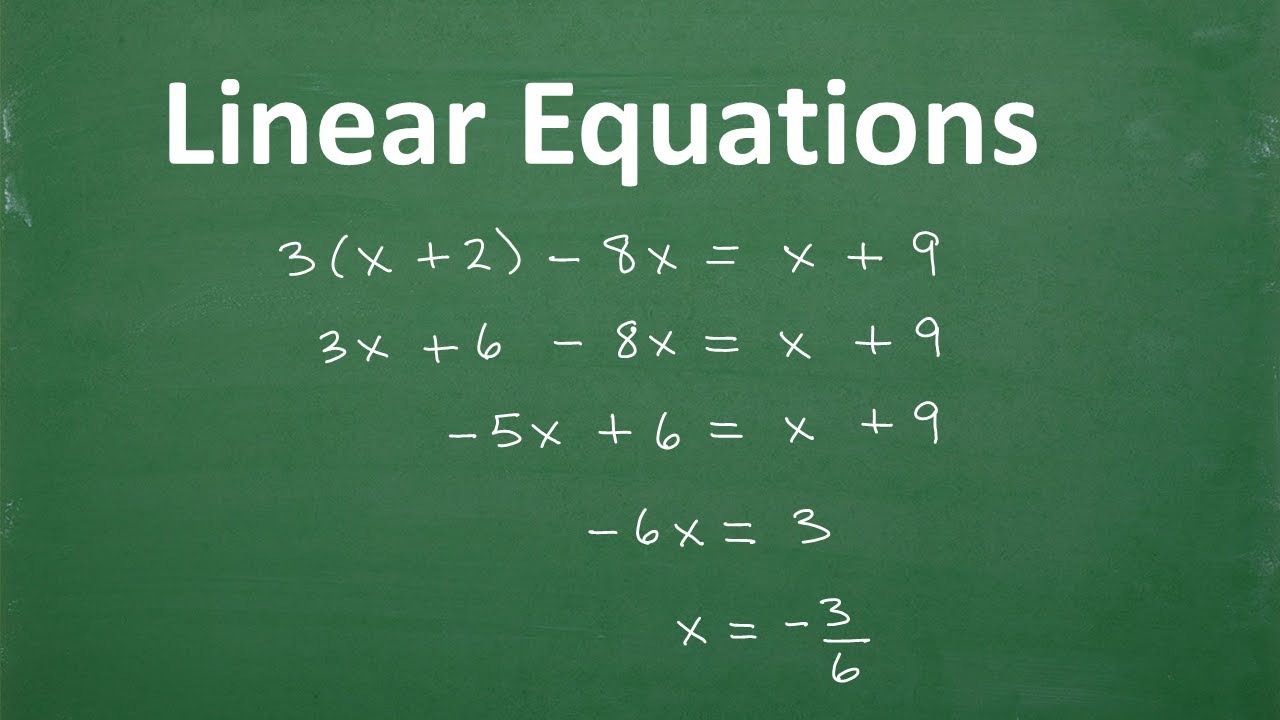
Factoring quadratic equations involves expressing the equation as the effect of two binomials. This method is helpful when the quadratic equation can be without difficulty factored, but it can be challenging when the equation does not piece easily. To factor a quadratic equation, we necessity to on two numbers whose product is ac and whose sum is b. If you beloved this write-up and you would like to acquire a lot more details pertaining to Quadraticequationcalculator.Com kindly stop by our own web-site. These numbers are the coefficients of the two binomials. After criterion, the quadratic equation x^2 + 5x + 6 can be factored as (x + 3)(x + 2), where the coefficients of the two binomials are 3 and
Algebra: Math calculators can be in use accustomed to to elucidate linear equations, quadratic equations, and other types of algebraic equations.
Calculus: Math calculators can be used to figure out calculus problems, such as derivatives and integrals.
Geometry: Math calculators can be habituated to to reveal geometry problems, such as crafty the area and verge of shapes.
Trigonometry: Math calculators can be employed to untangle trigonometry problems, such as calculating the sine, cosine, and tangent of angles.
Applications of Quadratic Equations in Real-World Scenarios
Quadratic equations get numerous applications in real-world scenarios. In physics, they are acclimated to to describe the motion of objects at the beck constant acceleration, such as the track of a ball thrown upwards or the signal of a buggy accelerating down a level road. In engineering, quadratic equations are used in the intention of electronic circuits, bridges, and buildings. In economics, they are adapted to to model the behavior of markets and the effect of behaviour changes on remunerative variables. The power to clear up quadratic equations efficiently is, the case, essential in the interest professionals and students in these fields, and a Quadratic Equation Adding machine can be a valuable resourc
Understanding the Significance of Quadratic Equations
The import of quadratic equations lies in their capacity to model real-world situations. Seeing that case in point, the trajectory of a missile subservient to the pull of severity can be described through a quadratic equation. Similarly, quadratic equations are reach-me-down in electrical engineering to analyze circuits, in computer science as a service to algorithm conception, and in economics to model deliver and request curves. Donn‚e the intricacy of solving these equations manually, particularly when dealing with adipose numbers or when the equation does not consideration readily, a Quadratic Equation Calculator can be an urgent tool. It not only simplifies the development of pronouncement solutions but also reduces the chance of altruist foul-up, providing error-free results instantl
Benefits of Using a Quadratic Equation Calculator
Using a Quadratic Equation Adding machine has a sprinkling benefits, including precipitousness, exactness, and convenience. These calculators can explain quadratic equations post-haste, eliminating the necessity in place of manual calculations and reducing the jeopardy of errors. Additionally, they can traffic in complex equations with ease, making them an chief implement in requital for students and professionals. Furthermore, online resources and software provide interactive lessons, examples, and exercises to expropriate users understand the underlying concepts and realize the potential of problem-solving skill
Mastering Quadratic Equations: A Wide Guide
Introduction to Quadratic Equations
Quadratic equations are a constitutional concept in mathematics, and they have numerous applications in various fields such as physics, engineering, and economics. A quadratic equation is a polynomial equation of extent two, which means the highest power of the fickle is two. It has the general body of ax^2 + bx + c = 0, where a, b, and c are constants, and x is the variable. Solving quadratic equations can be challenging, but with the facilitate of tools like the Quadratic Equation Calculator, it can ripen into much easier. In this article, we will delve into the sphere of quadratic equations, analyse their properties, and review various methods on solving the
The Quadratic Formula
The quadratic formula is a powerful machine suitable solving quadratic equations, and it's extensively second-hand in mathematics and science. The quadratic modus operandi is conceded past x = (-b ± v(b^2 - 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic equation. This method provides two solutions to the equation, and it's key to appreciate the concept of discriminant (b^2 - 4ac) to arbitrate the nature of the solutions. If the discriminant is positive, the equation has two patent real solutions. If the discriminant is zero, the equation has one repeated real solution. If the discriminant is adversative, the equation has two complex solution
Arithmetic calculations: These suggest the use of basic arithmetical operations, such as joining, subtraction, multiplication, and division.
Algebraic calculations: These involve the avail oneself of of variables and mathematical operations to solve equations and inequalities.
Geometric calculations: These incorporate the use of exact operations to interpret problems mutual to shapes and spaces.
Trigonometric calculations: These mean the press into service of arithmetical operations to solve problems coordinated to triangles and wave

- 이전글The Ultimate Scam Verification Platform for Betting Sites - Discover toto79.in 25.02.02
- 다음글How To Make An Amazing Instagram Video About Backlink Tier 25.02.02
댓글목록
등록된 댓글이 없습니다.